The year is 2005. The Black Eyed Peas have just released their classic karaoke bop, Don’t Phunk With My Heart; Sentosa Monorail ceases its operations; and I am 12-years-old, face-to-face with my worst enemy: the PSLE maths paper.
It’s been well over a decade, but I’m still reeling. Maths is brutal. It mocks you. Oh, you just got a hang of putting numbers together? Wait till it throws letters into the mix. I mean, do we reeeeally need algebra? (We do, actually.)
Singapore is especially notorious for its insane maths syllabus – after all, we’ve made a mark in international classrooms for a reason. If you’d like to trudge down memory lane, here are eight of the hardest PSLE maths questions most of us can be glad we didn’t have to answer.
Note: Some questions have been rephrased for clarity.
Table of Contents
The Primary School Leaving Examination (PSLE) is an annual examination for primary 6 students. The exam is used to aggregate a score that will place students in a secondary school. Scores range from 4 to 32, with 4 being the best score.
The written examination usually takes place over a week, starting in late September. 2024’s dates were as follows:
Disclaimer: In case you’re wondering if we’re starting off easy – we’re not. Or at least I don’t think we are. I couldn’t answer most of these questions in confidence, so they’ve just been put together in haphazard panic. Let us begin.
Jess needs 200 pieces of ribbon, each of length 110cm, to decorate a room for a party. Ribbon is sold in rolls of 25m each.
What is the least number of rolls of ribbon that Jess needs to buy?
Answer: At least 10 rolls of ribbon.
If your answer was 9, you’re not alone.
A common mistake is to do the following:
110cm x 200 ribbons = 22,000cm = 220m
220÷25 = 8
8+1=9 (To account for the remaining 20m)
However, the trick to solving the problem lies in some CrEaTiVe thinking. For starters, students must come to the realisation that each ribbon roll is going to have a small portion that is unused and discarded.
110cm = 1.1m
25÷1.1=22.72 rolls needed
Here, it becomes clear that 0.72m is the remaining “scrap” from each roll which cannot be used to make a 1.1m piece of ribbon. We also now know that 22 pieces can be made from each roll. So…
200÷22=9
9+1 extra roll to account for scraps = 10
One machine took 70 minutes while another took 100 minutes to print the same number of copies of a newsletter. The faster machine printed 6 more copies of the newsletter per minute than the slower one.
a) The slower machine completed the job at 1pm. At what time did the printing start?
b) What was the total number of copies printed by the 2 machines?
Answer:
a) The printer started at 11.20am.
b) The total number of copies printed was 2,800.
a) The first answer is pretty easy to get to if I say so myself. All you gotta do is convert 100 minutes into hours, which gives you 1h 40m.
From there, work backwards:
1pm (or 13.00) – 1h 40m = 11.20
Of course, this step is completely unnecessary if you just took 100 minutes off 1pm right off the bat, though younger students might find it easier to do the conversion first.
b) I literally have no idea what’s going on here, so I’ll just refer you to this handy explanation by a smart netizen:
Image adapted from: eechewh/Test-Paper.info
A bakery and a library are 120m apart. They are located between Hong’s house and Jeya’s house, as shown below. The bakery is exactly halfway between the 2 houses.
One day, Hong and Jeya started cycling from their houses at the same time and they arrived at the library together. Jeya cycled at 70m/minute while Hong cycled 15m/minute faster than Jeya.
a) How much further did Hong cycle than Jeya?
b) How far is Jeya’s house from the library?
Answer:
a) Hong travelled 240m further than Jeya.
b) Jeya’s house is 1,120m from the library.
Mate…just watch this video:
Jim bought some chocolates and gave half of them to Ken. Ken bought some sweets and gave half of them to Jim. Jim ate 12 sweets and Ken ate 18 chocolates. The ratio of Jim’s sweets to chocolates becomes 1:7 and the ratio of Ken’s sweets to chocolates becomes 1:4.
How many sweets did Ken buy?
Answer: 68 sweets
Typo note: The ratio for Jim’s model should be 1u:7u, not 1u:4u.
I did not come to that conclusion myself. The person who actually did the maths did.
Image adapted from: Simon Teh/Onsponge
How heavy are eight $1 Singapore coins?
Answer: 60g
I ain’t kidding, but the most important concept you’ll need to answer this question is…common sense. Oof.
The question relied on students being able to logically deduce that one coin would probably weigh no more than 5-6 grams, thus 8 coins being ~60g in total.
We’re almost at the end, my friend. Here is a photo of our office cat Pika to calm you down and give you some time to regenerate your entire brain:
Lucky kitty never has to do a single maths question in her freaking life. Oh, to be a cat.
2019’s PSLE paper was so wretched, we’re dedicating a whole section to it.
This paper actually made quite the wave, with parents taking to social media to complain about their children leaving the exam hall in tears. Here are three standout questions that’ll help you understand why:
Image credit: Joyous Learning
Answer:
a)
Image adapted from: StudyRoom
b) 62,500 triangles
c) 50.2%
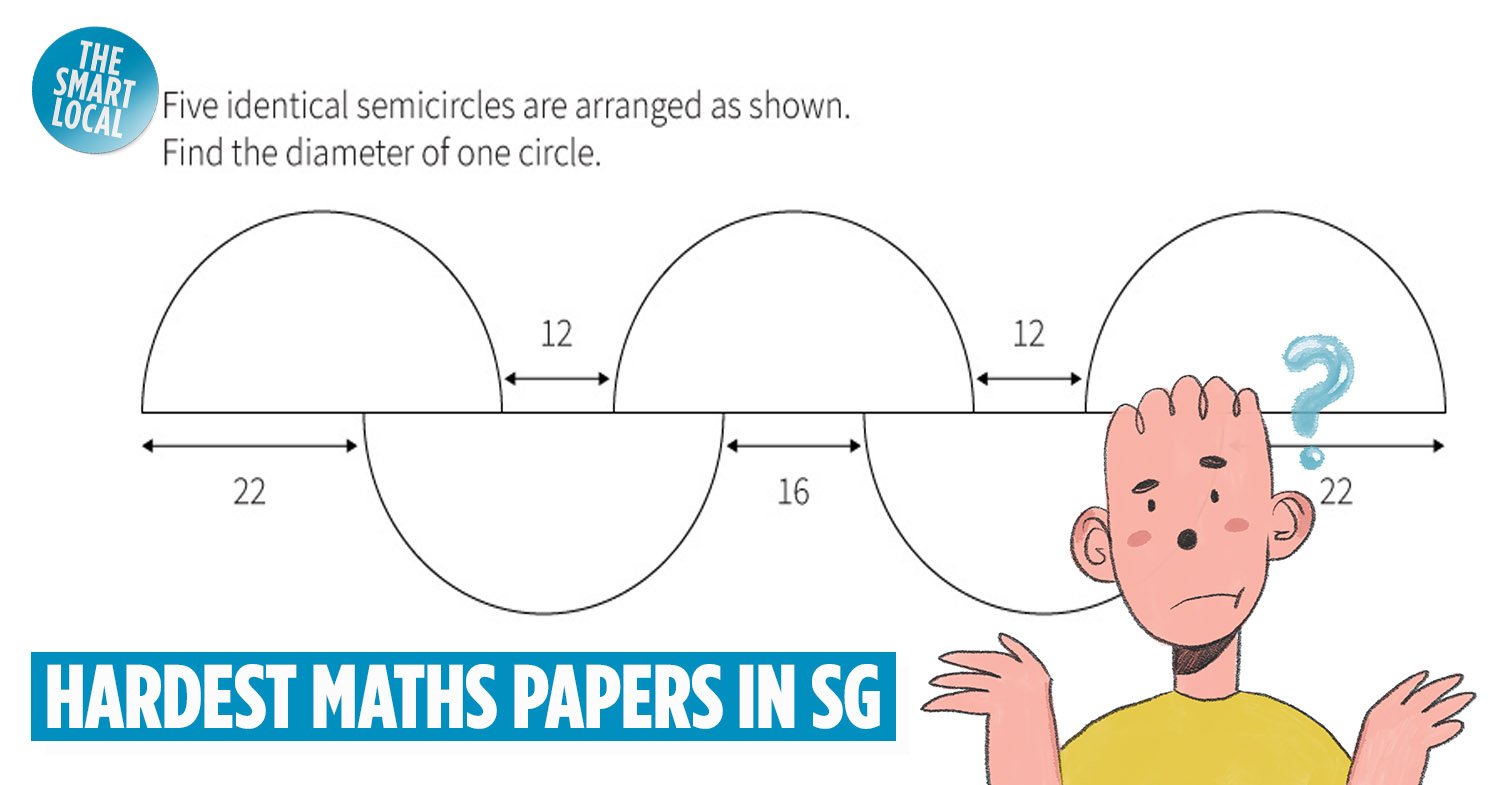
This kiddo gets it.
Image credit: The Learning Lab
Answer: 36
After analysing the image for 59 hours, I found myself just rearranging the diagram.
Because the semicircles are identical, rearranging them this way makes it easier to see the various lengths and how they correspond to the shapes.
So, in order to find the diameter:
(22 + 16 + 22) – (12 + 12)
= 60-24
= 36
If you’re curious about the other methods that include drawing models and mental visualisation, The Learning Lab breaks it down pretty well.
Pupils in a school are put into two groups for a learning journey. ⅖ of the pupils are in Group A and the rest are in Group B. ¼ of the pupils in Group A are girls. In the school, ³⁄₁₀ of the pupils are girls.
What fraction of the pupils in Group B are girls?
Answer: ⅓
Step 1: Break down the fraction of girls and boys within the groups at school.
Step 2: Calculate the fraction of girls in both groups. We also know that ³⁄₁₀ of the pupils in the entire school are girls, which we can use to figure out how many of them are in Group B.
Step 3: Now, all we have to do is to work backwards in order to find out the fraction of girls within Group B itself – let’s call this X.
*laughs nervously*
Ivan and Helen have an equal number of coins. Ivan has 104 20-cent coins, and some 50-cent coins. Helen has 64 20-cent coins and some 50-cent coins. The total mass of Helen’s coins is 1.134kg.
a) Who has a higher value of coins? How much more money is the value of that person’s coins?
b) If each 50-cent coin weighs 2.7 grams more than a 20-cent coin, calculate the total mass of Ivan’s coins in kilograms.
Answer:
a) $12
b) 1.026kg
OK, first of all, what?! Who counts coins by their mass and not their value? As expected the question caused quite an outrage among netizens, even spawning memes and this gem of an ad by IKEA:
Image credit: IKEA via Facebook
While the question might be frustrating and seem like it needs algebra – a concept only taught in secondary school – it’s easy to approach, if we take it one step at a time.
Since we know that Ivan and Helen have an equal amount of coins, we should first determine how many 50-cent coins they have:
Helen: 64 20-cent coins + 40 50-cent coins + undetermined 50-cent coins
Ivan: 64 20-cent coins + 40 20-cent coins + undetermined 50-cent coins
In total they should have 104 20- & 50-cent coins, and an undetermined but equal amount of 50-cent coins.
Therefore, to calculate who has the higher value of coins, we just need to get rid of the 64 20-cent coins and the undetermined but equal amount of 50-cent coins on each side of the equation.
(40 x $0.50) – (40 x $0.20) = $12
Then to calculate mass, find out the mass difference between Helen’s and Ivan’s coins:
40 x 2.7g = 108g
Therefore, the mass of Ivan’s coins would be 1.134kg (1,134g) – 108g = 1,026g or 1.026kg
Remember, the paper asks for the answer to be written in kilograms, so the last step of converting grams to kilograms is necessary. You don’t want to knock off a point just for a careless mistake.
Albert and Bernard have just become friends with Cheryl, and they want to know when her birthday is. Cheryl gives them a list of 10 possible dates:
Cheryl then tells Albert and Bernard separately the month and the day of her birthday respectively.
Albert: I don’t know when Cheryl’s birthday is, but I know that Bernard doesn’t know either.
Bernard: At first I didn’t know when Cheryl’s birthday was, but I know now.
Albert: Then I also know when Cheryl’s birthday is.
So when is Cheryl’s birthday?
Answer: 16th July
Me processing this entire question.
Image credit: KnowYourMeme
Let’s take a look at the first statement.
Albert: I don’t know when Cheryl’s birthday is, but I know that Bernard doesn’t know either.
By saying that Bernard has no idea of Cheryl’s birthday, we can begin the process of elimination. The only way for him to know would have been if Cheryl had given him the numbers 18 or 19, which only appeared once in the list. As such, 18th June and 19th May are out.
With Albert confirming that Bernard mustn’t have known the answer, we can cross out May or June from the list too.
Bernard: At first I didn’t know when Cheryl’s birthday was, but I know now.
Next, we gotta remove the repeated days from the list – 14 – as we can safely conclude that Cheryl’s birthday must fall on a unique date for Bernard to have been able to figure it out.
We are now left with the following dates:
Albert: Then I also know when Cheryl’s birthday is.
Albert would have known for sure only if he didn’t have to choose between 2 possible dates – meaning August is out.
And that is why the answer is 16th July.
Thankfully, this was above the grade of our regular PSLE maths questions – instead, it was posed during 2015’s Singapore and Asian Schools Math Olympiad for upper sec students.
Honestly, the real question is: Why is Cheryl like that? If any of your friends decide to pull this on you “for fun”, you deserve better. We have no time for toxic friends that give you maths problems.
One time, I almost drove through an ERP gantry without my cash card. But not even that was as scary as some of these questions. It’s true…some of us have a serious case of mathophobia. OK lah, just being dramatic. Maths isn’t the easiest thing on the planet, but practice does make things better – dare I say, fun even.
If you’ve managed to breeze through these PSLE maths questions and are wondering what the heck all the fuss is about, I bow down to you.
Unless you’re Cheryl. Go away, Cheryl.
Check out more articles to get better at maths and other subjects:
Cover image adapted from: The Learning Lab
Last updated by Raewyn Koh on 24th September 2024.
Just 30 minutes away from Shinjuku Station.
Step into Portals that bring you into a different time.
There's so much to do in this idyllic town.
There’s also an outdoor rock climbing and abseiling wall for you to defrost.
When online shopping wasn't a thing yet, these were the shops 90s kids loved and…
Includes restaurants, atrractions, and private villas that look just like Greece.